cubesraka.blogg.se

1. #BR ATOMIC RADIUS ZIP FILE#
2. #BR ATOMIC RADIUS FULL#
3. #BR ATOMIC RADIUS LICENSE#
4. #BR ATOMIC RADIUS DOWNLOAD#

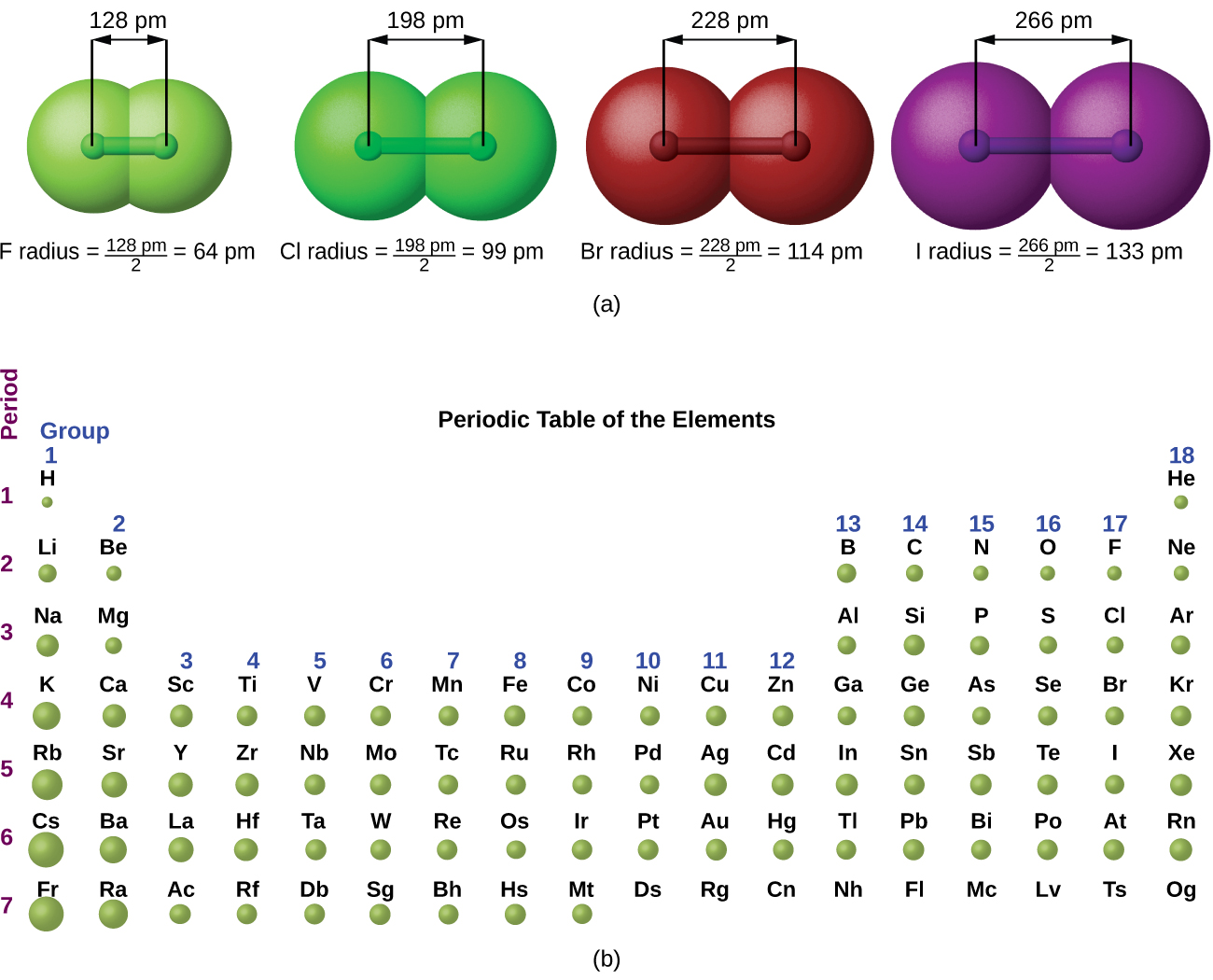
We assign half of this distance to each chlorine atom, giving chlorine a covalent atomic radius ( r cov) Half the distance between the nuclei of two like atoms joined by a covalent bond in the same molecule. For example, the internuclear distance in the diatomic Cl 2 molecule is known to be 198 pm. Because distances between the nuclei in pairs of covalently bonded atoms can be measured quite precisely, however, chemists use these distances as a basis for describing the approximate sizes of atoms. For similar reasons, the filled n = 2 shell in argon is located closer to the nucleus and has a lower energy than the n = 2 shell in neon.įigure 7.4 "Plots of Radial Probability as a Function of Distance from the Nucleus for He, Ne, and Ar" illustrates the difficulty of measuring the dimensions of an individual atom. The energy of the n = 1 shell also decreases tremendously (the filled 1 s orbital becomes more stable) as the nuclear charge increases.

#BR ATOMIC RADIUS FULL#

Consequently, the two electrons in the n = 1 shell experience nearly the full nuclear charge, resulting in a strong electrostatic interaction between the electrons and the nucleus. Because the 1 s 2 shell is closest to the nucleus, its electrons are very poorly shielded by electrons in filled shells with larger values of n. The peak for the filled n = 1 shell occurs at successively shorter distances for neon ( Z = 10) and argon ( Z = 18) because, with a greater number of protons, their nuclei are more positively charged than that of helium. Argon, with filled n = 1, 2, and 3 principal shells, has three peaks. In contrast, neon, with filled n = 1 and 2 principal shells, has two peaks. Because helium has only one filled shell ( n = 1), it shows only a single peak. Each peak in a given plot corresponds to the electron density in a given principal shell. In Ar, the 1 s electrons have a maximum at ≈2 pm, the 2 s and 2 p electrons combine to form a maximum at ≈18 pm, and the 3 s and 3 p electrons combine to form a maximum at ≈70 pm.įigure 7.4 "Plots of Radial Probability as a Function of Distance from the Nucleus for He, Ne, and Ar" also shows that there are distinct peaks in the total electron density at particular distances and that these peaks occur at different distances from the nucleus for each element. In Ne, the 1 s electrons have a maximum at ≈8 pm, and the 2 s and 2 p electrons combine to form another maximum at ≈35 pm (the n = 2 shell). In He, the 1 s electrons have a maximum radial probability at ≈30 pm from the nucleus.

#BR ATOMIC RADIUS ZIP FILE#

zip file containing this book to use offline, simply click here.įigure 7.4 Plots of Radial Probability as a Function of Distance from the Nucleus for He, Ne, and Ar

#BR ATOMIC RADIUS DOWNLOAD#

You can browse or download additional books there. More information is available on this project's attribution page.įor more information on the source of this book, or why it is available for free, please see the project's home page. Additionally, per the publisher's request, their name has been removed in some passages. However, the publisher has asked for the customary Creative Commons attribution to the original publisher, authors, title, and book URI to be removed. Normally, the author and publisher would be credited here. This content was accessible as of December 29, 2012, and it was downloaded then by Andy Schmitz in an effort to preserve the availability of this book.

#BR ATOMIC RADIUS LICENSE#

See the license for more details, but that basically means you can share this book as long as you credit the author (but see below), don't make money from it, and do make it available to everyone else under the same terms. This book is licensed under a Creative Commons by-nc-sa 3.0 license.